Graphing
Functions Where 0 < p < 1
When 0 < p < 1, the exponent is a fractional value that can be translated to a root. Since even roots have restrictions, some of these graphs will have a restricted domain. For graphs with a restricted domain, when k is positive the x and y-values will both approach positive infinity and will both stop at (0,0). When k is negative the x and y-values will both approach negative infinity and will both stop at (0,0).
Odd roots do not have restrictions, so when k is positive both x and y will approach positive infinity, and vice versa. When k is negative, as x approaches negative infinity y will approach positive infinity, and vice versa.
The graphs below represent, f(x) = 2x0.5 and g(x) = 2x0.2.
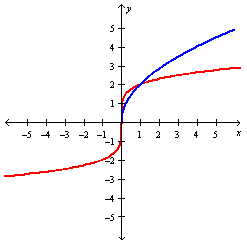
These graphs will be discussed in greater detail later in this unit.