Tables

Site:	Gladwin
Course:	Michigan Algebra I Sept. 2012
Book:	Tables

Printed by:	Guest user
Date:	Thursday, November 21, 2024, 8:59 AM

Description

Tables

Exponential Functions
Example 1
Example 2
- Example 2 Continued
Video Lesson
Guided Practice
Practice
- Answer Keys
Sources

Exponential Functions

Exponential functions are written in the form: y = ab^x, where b is the constant ratio and a is the initial value. By examining a table of ordered pairs, notice that as x increases by a constant value, the value of y increases by a common ratio. This is characteristic of all exponential functions.

In the table below notice that as the x-values increase by 1, the y-values double. In other words, to get from one y-value to the next, multiply by 2, therefore the common ratio is 2.

To find the equation that represents this table of values, substitute any ordered pair from the table into the equation, and solve for a.

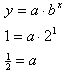
So the model for this data is:

Listen

Example 1

Find an exponential model for the table below.

TableEx1-1

Step 1. Find the common ratio for the table.

TableEx1-2

Step 2. Use an ordered pair to solve for the value of a.

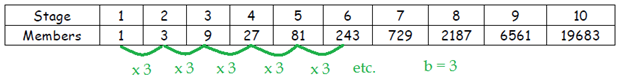
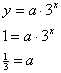
Step 3. Use the values of a and b to write an equation.

Example 2

Write an exponential model for the table below.

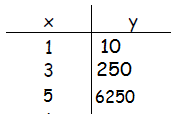
Step 1. Fill in the table to include the missing values for x.

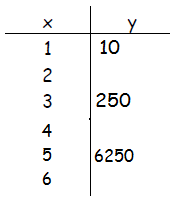
Example 2 Continued

Step 2. Find the value for b.

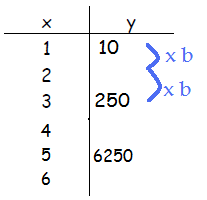
Since each value of y is moved 2 units for x, , or b² is required to move between two points. To determine the b -value, set up an equation and solve.

10â€¢b² = 250

b² = 25

b = 5

Step 3. Use an ordered pair to solve for the value of a.

For this example, use the point (1, 10)

TableEx2-5

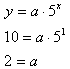
Step 4. Use the values of a and b to write an equation.

Video Lesson

To learn how to find models of exponential functions select the following link:

Modeling Exponential Functions #1

Guided Practice

To solidify your understanding of writing exponential models, visit the following link to Holt, Rinehart and Winston Homework Help Online. It provides examples, video tutorials and interactive practice with answers available. The Practice and Problem Solving section has two parts. The first part offers practice with a complete video explanation for the type of problem with just a click of the video icon. The second part offers practice with the solution for each problem only a click of the light bulb away.

Guided Practice